Mixed Number Meaning: Understanding The Basics And Beyond

So, you're here to dive deep into the world of mixed numbers, huh? Well, buckle up because we're about to take a journey through math that's both exciting and enlightening. Mixed number meaning might sound like something only math nerds care about, but trust me, it's more relevant than you think. Whether you're a student trying to ace your math test or just someone curious about how numbers work, this article has got you covered.

Mixed numbers are like the chameleons of the math world. They adapt, they change, and they're super useful in real-life situations. Think about splitting a pizza or dividing up a project at work—mixed numbers pop up everywhere! Understanding their meaning is key to mastering arithmetic, fractions, and even advanced math concepts.

But before we dive deeper, let's get one thing straight: mixed numbers aren't some alien concept. They're just a way of expressing numbers that are part whole and part fraction. It's like saying "I have 2 and a half apples" instead of writing it all out in fractions. Makes sense, right? Let's keep rolling and break it all down step by step.

• Chase Rice Wife The Untold Story Behind The Scenes

What Exactly is a Mixed Number?

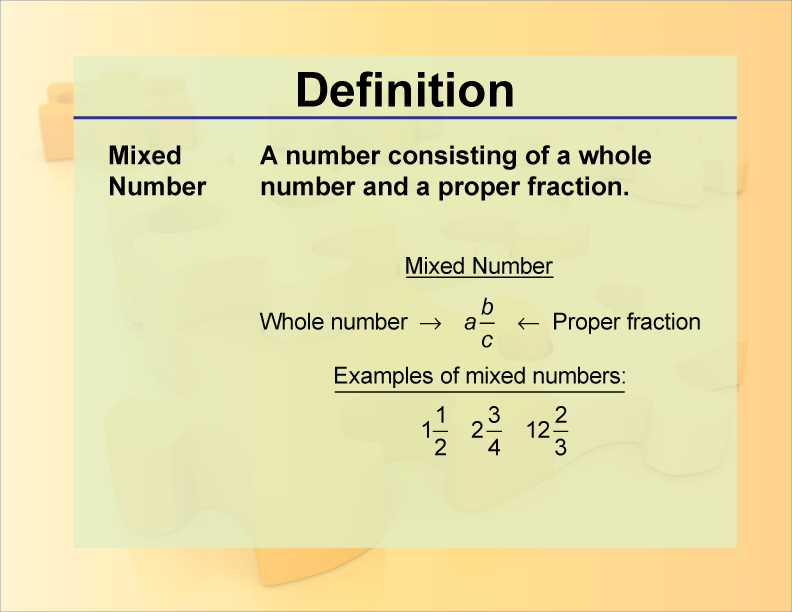
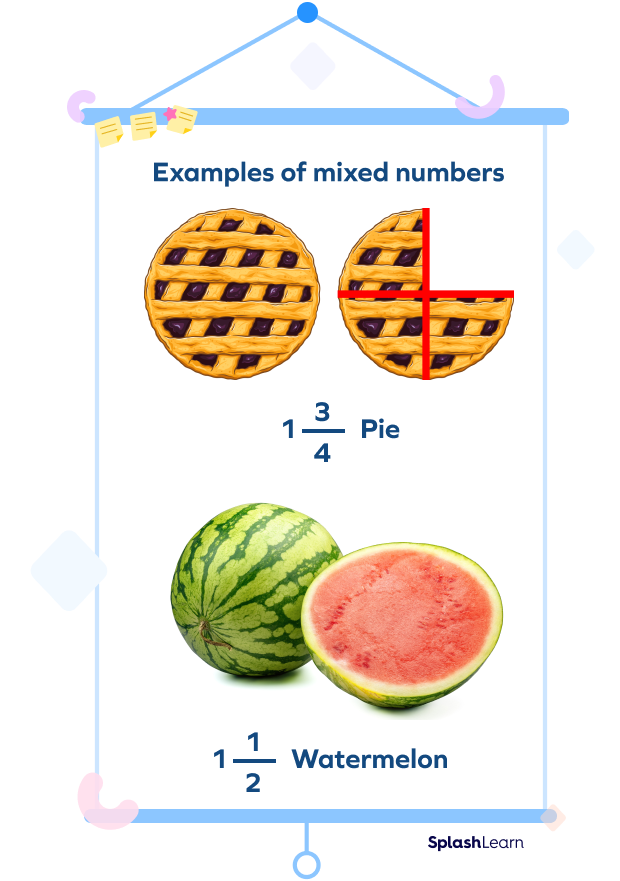
Alright, let's start with the basics. A mixed number is essentially a combo of a whole number and a fraction. It's like when you order a pizza and eat three full slices, but you're still hungry so you grab half of another slice. That's 3 and a half slices—or in math terms, 3 1/2. See? Easy peasy.

Here's the official definition: A mixed number consists of an integer (the whole number) and a proper fraction (a fraction where the numerator is smaller than the denominator). For example, 4 3/4 is a mixed number because 4 is the whole number, and 3/4 is the fraction.

Now, why do we need mixed numbers? Well, they make life simpler. Imagine trying to explain "I ate 3.75 slices of pizza" to your friends. Sounds kinda weird, right? Mixed numbers let us express numbers in a way that's easier to understand and work with.

• 324 Front Street Barnum Iowa Street View A Journey Through Time And Space

How Do Mixed Numbers Work?

Let's break it down further. When you see a mixed number like 5 2/3, what does it mean? It means you have 5 whole units, plus an additional 2/3 of a unit. It's like having 5 full apples and 2/3 of another apple. Simple, right?

Here’s how it works:

• The whole number tells you how many complete units you have.
• The fraction tells you how much of the next unit you have.

So, if you're dealing with 7 1/5, it means you have 7 full units and 1/5 of another unit. Easy enough, but there's more to explore!

Converting Mixed Numbers to Improper Fractions

Now, let's talk about converting mixed numbers to improper fractions. This is one of those math tricks that'll save you tons of time. An improper fraction is a fraction where the numerator is larger than or equal to the denominator. For example, 11/4 is an improper fraction.

Why convert? Sometimes, working with improper fractions is easier, especially when you're adding, subtracting, or multiplying fractions. Here's how you do it:

1. Multiply the whole number by the denominator of the fraction.
2. Add the result to the numerator of the fraction.
3. Write the new number over the original denominator.

For example, if you have 3 2/5:

• Multiply 3 (the whole number) by 5 (the denominator): 3 x 5 = 15.
• Add 2 (the numerator): 15 + 2 = 17.
• Write it as 17/5.

And just like that, you've got your improper fraction!

Converting Improper Fractions Back to Mixed Numbers

But wait, what if you need to go the other way? No problem. Converting improper fractions back to mixed numbers is just as simple. Here's how:

1. Divide the numerator by the denominator.
2. The quotient becomes the whole number.
3. The remainder becomes the numerator of the fraction, and the denominator stays the same.

Let's try it with 17/5:

• Divide 17 by 5: 17 ÷ 5 = 3 with a remainder of 2.
• The whole number is 3.
• The fraction is 2/5.

So, 17/5 becomes 3 2/5. Piece of cake, right?

Real-Life Applications of Mixed Numbers

Mixed numbers aren't just for math class. They pop up all over the place in everyday life. Here are a few examples:

Cooking and Baking

When you're following a recipe, you'll often see measurements like "2 1/2 cups of flour" or "1 3/4 teaspoons of salt." These are mixed numbers in action! Knowing how to work with them makes cooking a breeze.

Construction and DIY Projects

If you're building something, you'll probably need to measure lengths and widths. Mixed numbers help you express those measurements accurately. For example, "5 3/8 inches" is a lot clearer than "5.375 inches."

Time Management

Ever tried dividing your day into blocks of time? Mixed numbers can help with that too. If you spend 3 1/2 hours on one task and 2 1/4 hours on another, you can easily add them up to see how much time you've used.

Common Mistakes to Avoid

Even the best of us make mistakes when working with mixed numbers. Here are a few common ones to watch out for:

• Forgetting to simplify fractions: Always check if your fraction can be simplified after converting.
• Incorrectly adding or subtracting: Make sure you're working with the same denominators before you add or subtract.
• Mixing up numerators and denominators: Double-check your calculations to avoid swapping numbers.

By keeping these tips in mind, you'll avoid unnecessary headaches and get your math done right the first time.

Adding and Subtracting Mixed Numbers

Now, let's talk about operations. Adding and subtracting mixed numbers might seem tricky, but with a little practice, you'll be a pro in no time. Here's how:

Adding Mixed Numbers

Step 1: Add the whole numbers together.
Step 2: Add the fractions together.
Step 3: If the resulting fraction is improper, convert it back to a mixed number.

Example: 2 1/4 + 3 3/4

• Add the whole numbers: 2 + 3 = 5.
• Add the fractions: 1/4 + 3/4 = 4/4 = 1.
• Add the results: 5 + 1 = 6.

Final answer: 6.

Subtracting Mixed Numbers

Step 1: Subtract the whole numbers.
Step 2: Subtract the fractions.
Step 3: If the fraction part becomes negative, borrow from the whole number.

Example: 5 3/8 - 2 5/8

• Subtract the whole numbers: 5 - 2 = 3.
• Subtract the fractions: 3/8 - 5/8 = -2/8.
• Borrow 1 from the whole number: 3 - 1 = 2, and -2/8 + 8/8 = 6/8.

Final answer: 2 6/8 (or 2 3/4 after simplifying).

Multiplying and Dividing Mixed Numbers

Multiplying and dividing mixed numbers is a bit more advanced, but still totally doable. Here's how:

Multiplying Mixed Numbers

Step 1: Convert both mixed numbers to improper fractions.
Step 2: Multiply the numerators together and the denominators together.
Step 3: Simplify the result and convert back to a mixed number if needed.

Example: 2 1/2 x 3 1/3

• Convert to improper fractions: 2 1/2 = 5/2, 3 1/3 = 10/3.
• Multiply: (5/2) x (10/3) = 50/6.
• Simplify and convert: 50/6 = 8 2/6 = 8 1/3.

Dividing Mixed Numbers

Step 1: Convert both mixed numbers to improper fractions.
Step 2: Flip the second fraction (find its reciprocal) and multiply.
Step 3: Simplify the result and convert back to a mixed number if needed.

Example: 3 1/2 ÷ 1 1/4

• Convert to improper fractions: 3 1/2 = 7/2, 1 1/4 = 5/4.
• Flip the second fraction: 5/4 becomes 4/5.
• Multiply: (7/2) x (4/5) = 28/10.
• Simplify and convert: 28/10 = 2 8/10 = 2 4/5.

Tips for Mastering Mixed Numbers

Here are a few tips to help you become a mixed number master:

• Practice regularly: The more you work with mixed numbers, the easier they'll become.
• Use visual aids: Draw pictures or use fraction bars to help you visualize the numbers.
• Check your work: Always double-check your calculations to avoid mistakes.
• Stay calm: Math can be intimidating, but take it one step at a time and you'll get there.

Conclusion

So, there you have it—everything you need to know about mixed number meaning and how to work with them. Mixed numbers might seem tricky at first, but with a little practice, they'll become second nature. Whether you're cooking, building, or just solving math problems, mixed numbers are a valuable tool to have in your arsenal.

Now, it's your turn. Try solving a few mixed number problems on your own and see how far you've come. And don't forget to share this article with your friends and family—if they need a refresher on mixed numbers, this is the perfect guide!

Got any questions or comments? Drop them below, and let's keep the math conversation rolling. Until next time, happy calculating!

Table of Contents

• What Exactly is a Mixed Number?
• How Do Mixed Numbers Work?
• Converting Mixed Numbers to Improper Fractions
• Converting Improper Fractions Back to Mixed Numbers
• Real-Life Applications of Mixed Numbers
• Common Mistakes to Avoid
• Adding and Subtracting Mixed Numbers
• Multiplying and Dividing Mixed Numbers
• Tips for Mastering Mixed Numbers
• Conclusion