What's A Mixed Number? Understanding The Basics And Beyond

Alright, let's dive straight into the world of numbers, shall we? If you’ve ever stumbled upon the term “mixed number” and wondered, “What’s a mixed number, anyway?”—you’re definitely not alone. This concept is one of those math topics that might seem tricky at first but becomes super clear once you break it down. So, buckle up, because we’re about to demystify this number game for you.

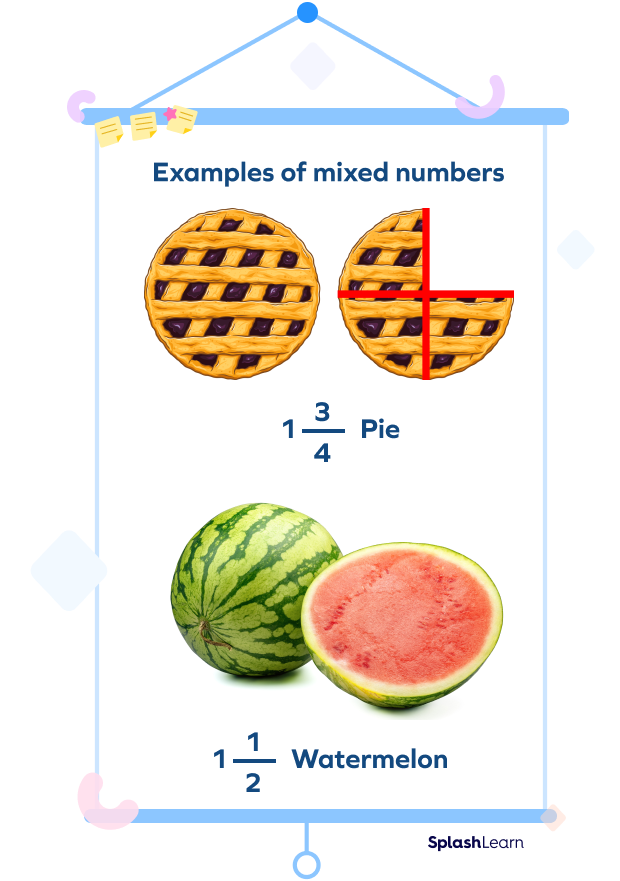
Mixed numbers are like the cool kids in the math world—they’re a combination of whole numbers and fractions working together as one. Imagine having a pizza that’s been cut into slices, and you’ve eaten part of it. How do you describe what’s left? That’s where mixed numbers come in handy. They’re practical, useful, and honestly, not as intimidating as they sound.

Now, why should you care about mixed numbers? Well, whether you’re a student trying to ace your math exams, a parent helping your kid with homework, or just someone curious about how numbers work, understanding mixed numbers can open up a whole new world of problem-solving skills. Let’s get started!

• Home Value Estimator Zillow Your Ultimate Guide To Understanding Zillows Zestimate

What Exactly Is a Mixed Number?

A mixed number is basically a number that has two parts: a whole number and a fraction. Think of it like this—it’s a way to express quantities that aren’t entirely whole but also aren’t just fractions. For example, if you have 3 whole apples and half an apple, you can write it as 3 1/2. See? Not so scary, right?

Mixed numbers are often used in everyday situations, like cooking, measuring, and dividing things. They’re also super important in math because they help us work with numbers that aren’t perfectly whole. You’ll see them pop up in all kinds of problems, from basic arithmetic to advanced algebra.

Breaking Down the Components

• Whole Number: This is the “solid” part of the mixed number. It represents how many complete units you have.
• Fraction: This is the “partial” part of the mixed number. It shows how much of the next whole unit you have.

For instance, in the mixed number 4 3/4, the whole number is 4, and the fraction is 3/4. Together, they tell you that you have 4 full units and 3 out of 4 parts of the next unit.

• Daryl Mitchell The Rising Star In International Cricket

Why Are Mixed Numbers Important?

Mixed numbers are more than just a math concept—they’re a practical tool for real-life situations. Let’s say you’re baking a cake, and the recipe calls for 2 1/2 cups of flour. How do you measure that? By using a mixed number! Or maybe you’re splitting a bill with friends, and each person owes $15 1/3. Mixed numbers make these kinds of calculations easier to understand.

They’re also crucial in fields like engineering, construction, and finance, where precision matters. Imagine building a house and needing to cut a piece of wood that’s 7 1/8 inches long. Without mixed numbers, that kind of measurement would be a lot harder to handle.

Where Do Mixed Numbers Show Up?

• Cooking and Baking
• Construction and Carpentry
• Finance and Accounting
• Science and Engineering
• Everyday Measurements

So, whether you’re a chef, a carpenter, or just someone trying to figure out how much paint you need for a room, mixed numbers are your secret weapon.

How Do You Write a Mixed Number?

Writing a mixed number is pretty straightforward. You simply combine a whole number with a fraction. The whole number goes first, followed by the fraction. For example:

5 2/3

This means you have 5 whole units and 2 out of 3 parts of the next unit. Easy peasy, right?

Tips for Writing Mixed Numbers

• Make sure the fraction part is always less than 1. If it’s greater than or equal to 1, you’ll need to convert it to a mixed number.
• Keep the fraction in its simplest form. For example, instead of writing 4 6/8, simplify it to 4 3/4.

By following these tips, you’ll ensure your mixed numbers are clear and easy to understand.

Converting Between Mixed Numbers and Improper Fractions

One of the coolest things about mixed numbers is that you can easily convert them to improper fractions and vice versa. Why would you want to do this? Well, sometimes it’s easier to work with improper fractions when solving math problems. Plus, it’s just another way to think about numbers.

From Mixed Numbers to Improper Fractions

Here’s how you do it:

1. Multiply the whole number by the denominator of the fraction.
2. Add the result to the numerator of the fraction.
3. Write the sum as the new numerator, keeping the same denominator.

For example, let’s convert 3 1/4 to an improper fraction:

• Multiply 3 × 4 = 12
• Add 12 + 1 = 13
• Write 13/4

Voilà! You’ve got your improper fraction.

From Improper Fractions to Mixed Numbers

To go the other way:

1. Divide the numerator by the denominator.
2. The quotient becomes the whole number.
3. The remainder becomes the numerator of the fraction, and the denominator stays the same.

For example, let’s convert 11/3 to a mixed number:

• Divide 11 ÷ 3 = 3 remainder 2
• Write 3 2/3

Boom! You’ve got your mixed number.

Adding and Subtracting Mixed Numbers

Adding and subtracting mixed numbers might sound tricky, but with a little practice, it’s totally doable. The key is to break it down into smaller steps.

Adding Mixed Numbers

Here’s the process:

1. Add the whole numbers together.
2. Add the fractions together.
3. If the resulting fraction is improper, convert it to a mixed number and add the whole number part to the sum.

For example, let’s add 2 1/2 and 1 3/4:

• Whole numbers: 2 + 1 = 3
• Fractions: 1/2 + 3/4 = 5/4
• Convert 5/4 to 1 1/4
• Add 3 + 1 = 4 1/4

And there you have it!

Subtracting Mixed Numbers

Subtraction works similarly:

1. Subtract the whole numbers.
2. Subtract the fractions.
3. If the second fraction is larger, borrow from the whole number part.

For example, let’s subtract 3 1/4 from 5 3/4:

• Whole numbers: 5 - 3 = 2
• Fractions: 3/4 - 1/4 = 2/4
• Simplify 2/4 to 1/2
• Result: 2 1/2

Simple, right?

Multiplying and Dividing Mixed Numbers

Multiplying and dividing mixed numbers might seem a bit more challenging, but don’t worry—it’s all about converting them to improper fractions first.

Multiplying Mixed Numbers

Here’s how you do it:

1. Convert both mixed numbers to improper fractions.
2. Multiply the numerators together and the denominators together.
3. Convert the result back to a mixed number if needed.

For example, let’s multiply 2 1/2 by 1 3/4:

• Convert 2 1/2 to 5/2 and 1 3/4 to 7/4
• Multiply 5 × 7 = 35 and 2 × 4 = 8
• Result: 35/8
• Convert 35/8 to 4 3/8

And you’re done!

Dividing Mixed Numbers

Division works similarly:

1. Convert both mixed numbers to improper fractions.
2. Flip the second fraction (take its reciprocal).
3. Multiply the numerators and denominators.
4. Convert the result back to a mixed number if needed.

For example, let’s divide 3 1/2 by 1 1/4:

• Convert 3 1/2 to 7/2 and 1 1/4 to 5/4
• Flip 5/4 to 4/5
• Multiply 7 × 4 = 28 and 2 × 5 = 10
• Result: 28/10
• Convert 28/10 to 2 8/10, then simplify to 2 4/5

There you go!

Common Mistakes to Avoid

Even the best of us make mistakes when working with mixed numbers. Here are a few common ones to watch out for:

• Forgetting to simplify fractions.
• Not converting improper fractions back to mixed numbers.
• Mixing up numerators and denominators during multiplication or division.

The good news is that with practice, you’ll get the hang of it in no time.

How to Avoid These Mistakes

• Double-check your work.
• Use scratch paper to keep track of your calculations.
• Practice regularly to build confidence.

Remember, practice makes perfect!

Real-Life Examples of Mixed Numbers

Let’s take a look at some real-life scenarios where mixed numbers come in handy:

Cooking and Baking

Recipes often use mixed numbers for measurements. For example:

• 2 1/2 cups of flour
• 1 3/4 teaspoons of salt

Understanding mixed numbers helps you follow recipes accurately.

Construction and Carpentry

Measurements in construction are frequently expressed as mixed numbers:

• 7 1/8 inches
• 12 3/4 feet

Knowing how to work with mixed numbers ensures precise cuts and builds.

Finance and Accounting

Financial calculations sometimes involve mixed numbers:

• $15 1/3 per hour
• 6 1/2% interest rate

Being comfortable with mixed numbers can help you make informed financial decisions.

Conclusion: Embrace the Power of Mixed Numbers

So, there you have it—a complete guide to understanding what mixed numbers are and how they work. From basic definitions to advanced operations, we’ve covered everything you need to know to master this essential math concept.

Remember, mixed numbers aren’t just for math class—they’re a valuable tool for everyday life. Whether you’re cooking, building, or managing finances, mixed numbers can help you solve problems more effectively.

Now it’s your turn! Take what you’ve learned and start practicing. Leave a comment below to share your thoughts or ask any questions. And don’t forget to check out our other articles for more math tips and tricks. Happy calculating!

Table of Contents

• What Exactly Is a Mixed Number?