What Is Mixed Number? A Comprehensive Guide To Understanding Mixed Fractions

**Hey there, math enthusiasts! If you're scratching your head over the term "mixed number" and wondering what on earth it means, don't worry—you're not alone. Mixed numbers are a fascinating part of the math world that combines whole numbers with fractions. Sounds intriguing, right? Let’s dive into this concept together and unravel the mystery behind mixed numbers.**

Mixed numbers are more common than you might think. Whether you're measuring ingredients for a recipe or splitting pizza slices with friends, chances are you've encountered a mixed number in your everyday life. It’s like a hybrid of whole numbers and fractions that makes math a little more relatable and practical.

In this article, we’ll explore everything you need to know about mixed numbers. From their definition and examples to how they’re used in real-life scenarios, we’ve got you covered. So, grab your favorite snack, sit back, and let’s make math fun again!

• Andrew Deluca A Deep Dive Into The Extraordinary Life Achievements And Legacy

Table of Contents

• What is Mixed Number?
• Parts of a Mixed Number
• Examples of Mixed Numbers
• Converting Mixed Numbers
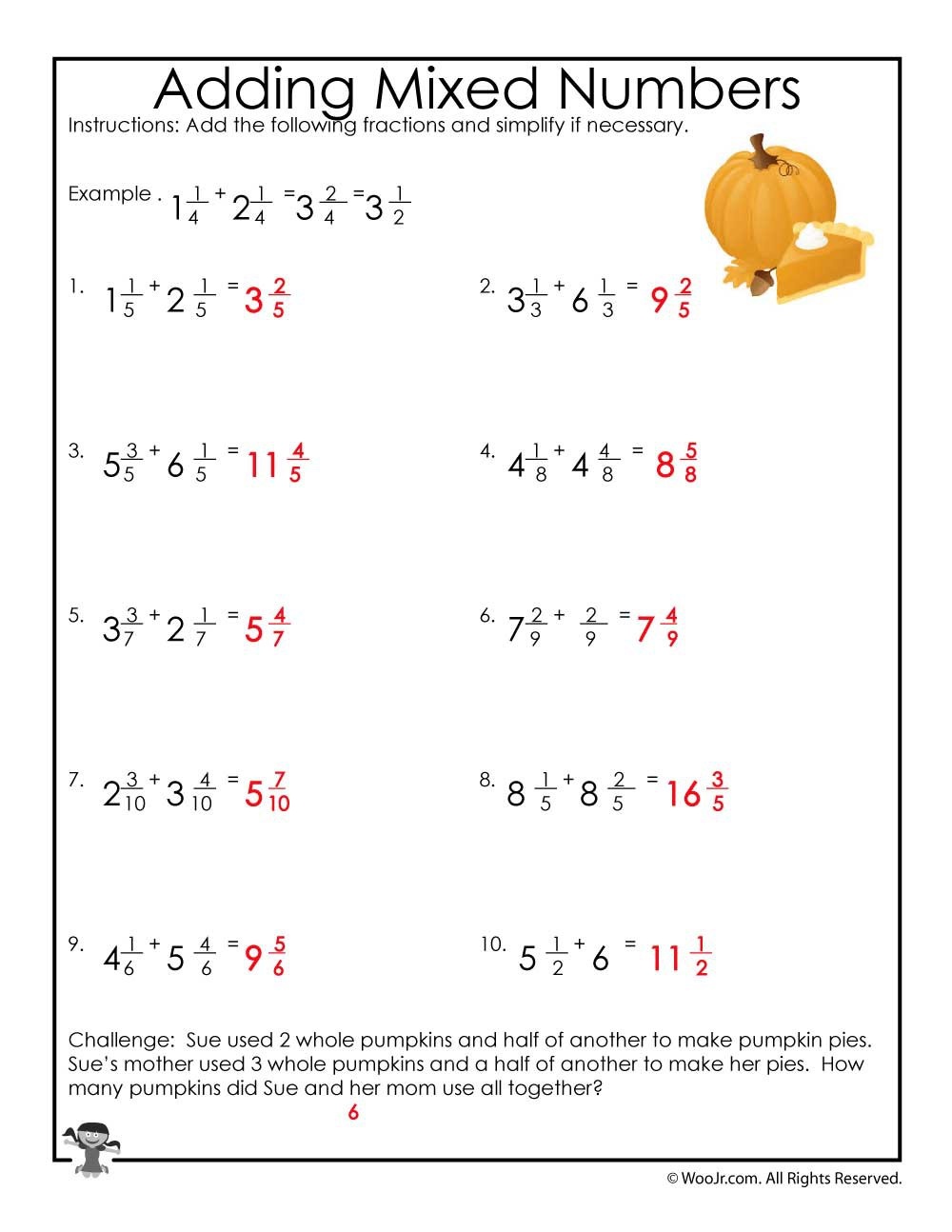
• Adding Mixed Numbers
• Subtracting Mixed Numbers
• Multiplying Mixed Numbers
• Dividing Mixed Numbers
• Real-Life Applications of Mixed Numbers
• Tips for Learning Mixed Numbers

What is Mixed Number?

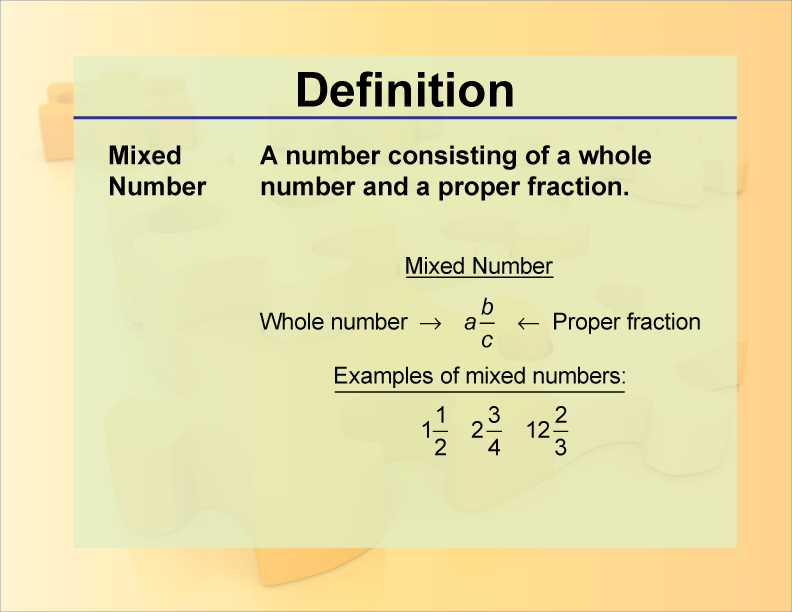
A mixed number, also known as a mixed fraction, is a combination of a whole number and a proper fraction. Think of it like this: it’s when you have a full pizza and a few slices left over. The whole number represents the complete pizzas, while the fraction represents the leftover slices. For example, 2 1/4 is a mixed number where "2" is the whole number and "1/4" is the fraction.

Mixed numbers are super useful because they help us express quantities that are more than a whole but less than the next whole number. They’re like the Goldilocks of numbers—not too big, not too small, just right!

Let’s break it down further: mixed numbers are written in the form of "a b/c," where "a" is the whole number, "b" is the numerator of the fraction, and "c" is the denominator. Easy peasy, right?

• Chase Rice Wife The Untold Story Behind The Scenes

Parts of a Mixed Number

Every mixed number has three main components: the whole number, the numerator, and the denominator. Here’s a quick breakdown:

• Whole Number: This is the integer part of the mixed number. It represents how many complete units you have.
• Numerator: This is the top number of the fraction. It tells you how many parts you have out of the total.
• Denominator: This is the bottom number of the fraction. It shows how many equal parts make up one whole.

For instance, in the mixed number 3 2/5:

• 3 is the whole number.
• 2 is the numerator.
• 5 is the denominator.

Now that we’ve got the basics down, let’s move on to some examples!

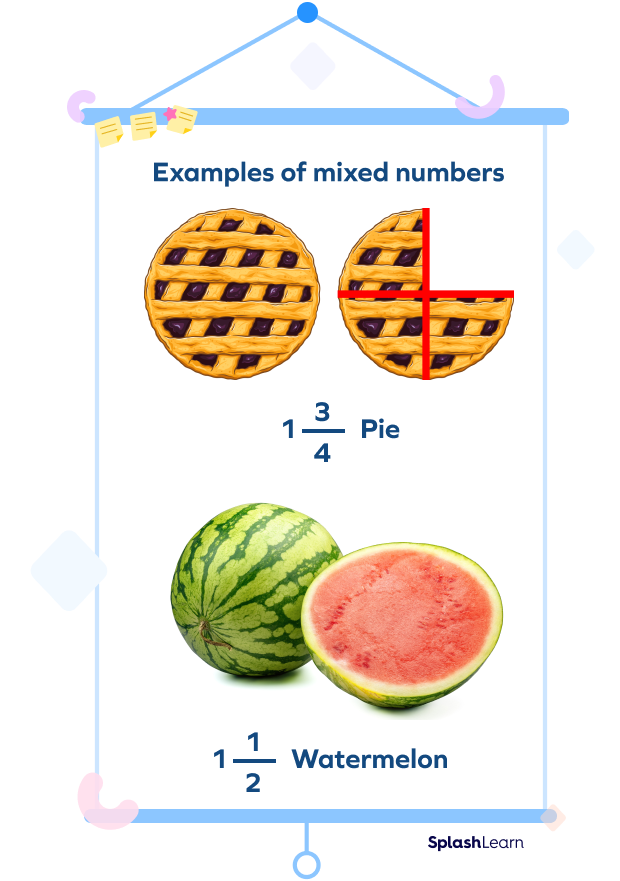
Examples of Mixed Numbers

Here are a few examples of mixed numbers to help you visualize them better:

• 1 1/2: One whole and one-half.
• 4 3/8: Four wholes and three-eighths.
• 7 5/6: Seven wholes and five-sixths.
• 9 2/3: Nine wholes and two-thirds.

See how they work? Each example combines a whole number with a fraction to represent quantities that aren’t whole numbers.

Why Are Mixed Numbers Important?

Mixed numbers are important because they allow us to express quantities that are greater than one but not whole. They’re particularly useful in everyday situations where precision matters, like cooking, construction, and even budgeting.

Converting Mixed Numbers

Converting mixed numbers is an essential skill in math. You can convert a mixed number into an improper fraction or vice versa. Here’s how it works:

Converting Mixed Numbers to Improper Fractions

To convert a mixed number to an improper fraction, follow these steps:

1. Multiply the whole number by the denominator.
2. Add the result to the numerator.
3. Write the sum as the new numerator, keeping the same denominator.

For example, let’s convert 3 1/4:

• Multiply 3 (whole number) by 4 (denominator): 3 × 4 = 12.
• Add 1 (numerator): 12 + 1 = 13.
• The improper fraction is 13/4.

Converting Improper Fractions to Mixed Numbers

To convert an improper fraction to a mixed number, follow these steps:

1. Divide the numerator by the denominator.
2. The quotient becomes the whole number.
3. The remainder becomes the numerator of the fraction, and the denominator stays the same.

For example, let’s convert 17/5:

• Divide 17 by 5: 17 ÷ 5 = 3 remainder 2.
• The whole number is 3, and the fraction is 2/5.
• The mixed number is 3 2/5.

Adding Mixed Numbers

Adding mixed numbers might seem tricky at first, but with a little practice, it becomes second nature. Here’s how you do it:

1. Add the whole numbers together.
2. Add the fractions together. If the fractions have different denominators, find a common denominator first.
3. If the resulting fraction is improper, convert it to a mixed number and add the whole number part to the sum.

For example, let’s add 2 1/3 and 1 2/3:

• Add the whole numbers: 2 + 1 = 3.
• Add the fractions: 1/3 + 2/3 = 3/3 = 1.
• Add the results: 3 + 1 = 4.

The final answer is 4.

Subtracting Mixed Numbers

Subtracting mixed numbers is similar to adding them. Here’s the process:

1. Subtract the whole numbers.
2. Subtract the fractions. If the second fraction is larger than the first, borrow from the whole number.
3. Simplify the result if necessary.

For example, let’s subtract 3 1/4 from 5 3/4:

• Subtract the whole numbers: 5 - 3 = 2.
• Subtract the fractions: 3/4 - 1/4 = 2/4 = 1/2.
• The final answer is 2 1/2.

Multiplying Mixed Numbers

Multiplying mixed numbers is a bit different. First, convert the mixed numbers to improper fractions, then multiply the numerators and denominators separately. Finally, simplify the result if needed.

For example, let’s multiply 2 1/2 and 3 1/3:

• Convert to improper fractions: 2 1/2 = 5/2, 3 1/3 = 10/3.
• Multiply the fractions: (5 × 10) / (2 × 3) = 50/6.
• Simplify: 50/6 = 8 2/6 = 8 1/3.

The final answer is 8 1/3.

Dividing Mixed Numbers

Dividing mixed numbers is similar to multiplying them. Convert the mixed numbers to improper fractions, then multiply the first fraction by the reciprocal of the second fraction. Simplify the result if needed.

For example, let’s divide 3 1/2 by 1 1/4:

• Convert to improper fractions: 3 1/2 = 7/2, 1 1/4 = 5/4.
• Multiply by the reciprocal: (7/2) × (4/5) = 28/10.
• Simplify: 28/10 = 2 8/10 = 2 4/5.

The final answer is 2 4/5.

Real-Life Applications of Mixed Numbers

Mixed numbers aren’t just a math concept—they have real-world applications. Here are a few examples:

• Cooking: Recipes often require measurements like 1 1/2 cups of flour or 2 3/4 teaspoons of sugar.
• Construction: Builders use mixed numbers to measure materials, such as 5 1/2 inches of lumber.
• Finance: Budgets may include expenses like $300 1/4 for groceries or $1,250 1/2 for rent.

Understanding mixed numbers can make these tasks easier and more accurate.

Tips for Learning Mixed Numbers

Mastering mixed numbers takes practice, but with the right approach, you can do it. Here are some tips:

• Practice converting between mixed numbers and improper fractions.
• Use visual aids like fraction bars or number lines to understand the concept better.
• Solve real-life problems involving mixed numbers to see their practical applications.
• Work on math problems regularly to build confidence and fluency.

Remember, practice makes perfect. The more you work with mixed numbers, the more comfortable you’ll become with them.

Final Thoughts

Mixed numbers might seem intimidating at first, but once you understand how they work, they’re actually pretty straightforward. They’re a powerful tool for expressing quantities that aren’t whole numbers, and they have countless real-world applications.

So, the next time you encounter a mixed number, don’t panic! Use the tips and techniques we’ve discussed, and you’ll be solving mixed number problems like a pro in no time. And hey, if you found this article helpful, don’t forget to share it with your friends or leave a comment below. Let’s make math fun together!