What Is The Meaning Of Mixed Number? A Simple Breakdown You'll Love!

Have you ever stumbled upon the term "mixed number" and wondered what it actually means? Don’t worry; you’re not alone! Mixed numbers are one of those math concepts that might sound intimidating at first, but once you break it down, it’s as easy as pie—or maybe even easier! If you’ve been scratching your head over this concept, we’ve got your back. In this article, we’ll dive deep into the world of mixed numbers, breaking them down step by step so you can finally understand them like a pro.

Let’s face it: math isn’t everyone’s favorite subject, but when it comes to mixed numbers, there’s no need to panic. This concept is actually pretty straightforward once you get the hang of it. Whether you’re a student trying to ace your math homework or an adult brushing up on your skills, this article will guide you through everything you need to know about mixed numbers. So grab your favorite snack, and let’s get started!

By the end of this read, you’ll not only know what mixed numbers are but also how to work with them in real-life situations. We’ll cover everything from definitions to practical applications, so stick around for some seriously useful insights!

• How Tall Hugh Jackman Unveiling The Wolverinersquos Height And More

What Exactly is a Mixed Number?

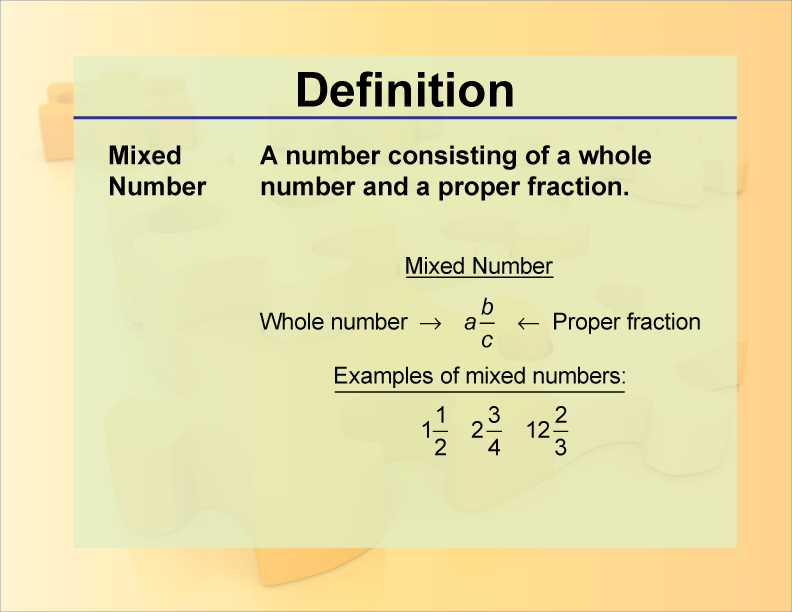
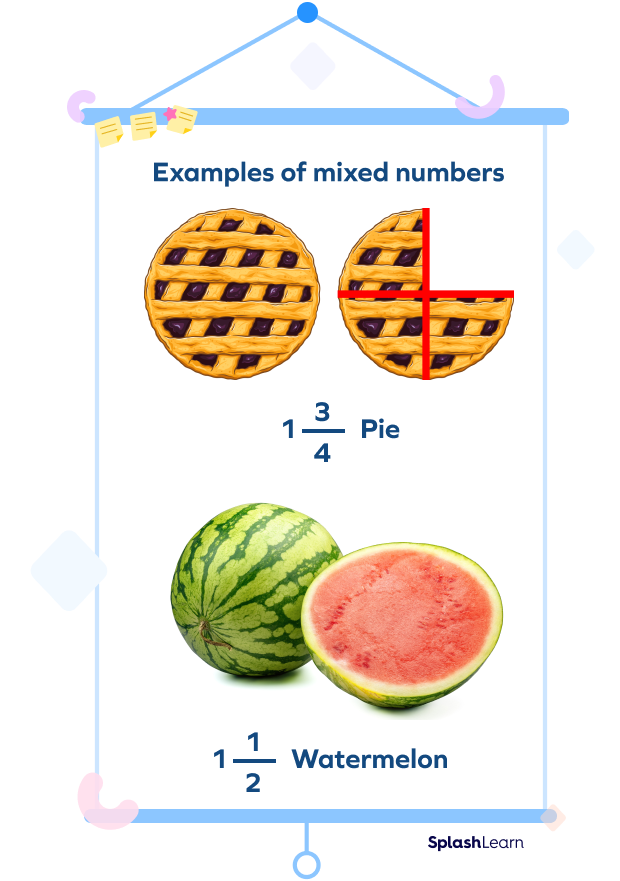
Alright, let’s cut to the chase. A mixed number is basically a combination of two things: a whole number and a fraction. Think of it like ordering a pizza—you’ve got a couple of full pizzas, and then there’s that one slice left over. That leftover slice is your fraction, while the full pizzas are your whole numbers. Together, they form what we call a mixed number.

For example, if you have 2 full pizzas and 3 slices from another pizza (out of 8 total slices), you’d write it as 2 3/8. See? Not so scary after all! This kind of number is super common in everyday life, from cooking recipes to splitting bills with friends.

Why Do Mixed Numbers Matter?

Mixed numbers aren’t just some abstract concept from your math textbook; they’re actually super useful in real life. Imagine you’re following a recipe that calls for 1 1/2 cups of flour. Or maybe you’re dividing up a pizza among a group of friends. Mixed numbers make these kinds of calculations easier to understand and work with.

• Tom Guiry The Rising Star Who Stole Our Hearts In Hollywood

They’re also essential in fields like construction, engineering, and even finance. For instance, if a carpenter needs to cut a piece of wood that’s 3 1/4 feet long, knowing how to handle mixed numbers can save time and materials. It’s all about precision, and mixed numbers help us achieve exactly that.

Where Do Mixed Numbers Appear in Daily Life?

• Cooking and Baking: Recipes often use measurements like 2 1/2 cups or 3 3/4 teaspoons.
• DIY Projects: Measuring lengths, widths, or heights frequently involves mixed numbers.
• Shopping: Discounts or sales might involve fractions, like "Buy 2 1/2 dozen donuts for $10!"
• Travel: Gas mileage or distance calculations sometimes require working with mixed numbers.

Breaking Down the Components of a Mixed Number

Now that we know what mixed numbers are, let’s break them down into their parts. Every mixed number consists of two main components: the whole number and the fraction. The whole number tells you how many complete units you have, while the fraction represents the leftover part.

Take 4 2/5, for instance. Here, 4 is the whole number, meaning you have four full units. The 2/5 is the fraction, indicating that you have two-fifths of another unit. Simple, right? Let’s explore each component in more detail.

Understanding Whole Numbers

Whole numbers are pretty self-explanatory—they’re the numbers we use to count things like apples, books, or people. They include 0, 1, 2, 3, and so on. In mixed numbers, the whole number gives you a sense of how many full units you’re dealing with.

For example, in 6 1/3, the whole number is 6, meaning you have six complete items. Easy peasy!

Getting a Grip on Fractions

Fractions are where things get a little more interesting. A fraction represents a part of a whole, usually written as a numerator over a denominator. The numerator tells you how many parts you have, while the denominator shows how many parts make up the whole.

In the mixed number 7 3/8, the fraction is 3/8. This means you have three-eighths of another unit in addition to the seven full units. Fractions might seem tricky at first, but with a bit of practice, they’ll become second nature.

How to Convert Mixed Numbers to Improper Fractions

Sometimes, it’s easier to work with improper fractions instead of mixed numbers. An improper fraction is a fraction where the numerator is greater than or equal to the denominator. To convert a mixed number to an improper fraction, follow these simple steps:

1. Multiply the whole number by the denominator of the fraction.
2. Add the numerator to the result.
3. Write the sum as the new numerator, keeping the same denominator.

For example, let’s convert 3 2/5 to an improper fraction:

• Multiply 3 (whole number) by 5 (denominator): 3 × 5 = 15.
• Add 2 (numerator): 15 + 2 = 17.
• Write the result as 17/5.

And just like that, you’ve got your improper fraction!

Converting Improper Fractions Back to Mixed Numbers

Going the other way is just as easy. To convert an improper fraction back to a mixed number, divide the numerator by the denominator. The quotient becomes the whole number, and the remainder becomes the numerator of the fraction. The denominator stays the same.

Let’s try converting 11/4 to a mixed number:

• Divide 11 (numerator) by 4 (denominator): 11 ÷ 4 = 2 remainder 3.
• The whole number is 2, and the fraction is 3/4.
• So, the mixed number is 2 3/4.

See? Not so bad!

Why Bother Converting?

Converting between mixed numbers and improper fractions can make certain math problems much simpler. For example, adding or subtracting fractions becomes a lot easier when everything is in the same format. Plus, it’s always good to have multiple tools in your math toolbox!

Adding and Subtracting Mixed Numbers

Adding and subtracting mixed numbers might sound tricky, but with a little practice, you’ll be doing it like a pro. The key is to break the problem into manageable steps:

1. Add or subtract the whole numbers first.
2. Then, add or subtract the fractions.
3. If the fractions don’t have the same denominator, find a common denominator before proceeding.

Let’s try an example: Add 2 1/3 and 1 2/3.

• Add the whole numbers: 2 + 1 = 3.
• Add the fractions: 1/3 + 2/3 = 3/3, which simplifies to 1.
• Combine the results: 3 + 1 = 4.

So, 2 1/3 + 1 2/3 = 4. Piece of cake!

Multiplying and Dividing Mixed Numbers

Multiplying and dividing mixed numbers is a bit different, but don’t worry—it’s still manageable. The first step is always to convert the mixed numbers to improper fractions. From there, follow the standard rules for multiplying or dividing fractions.

For example, let’s multiply 2 1/2 by 3 1/3:

• Convert 2 1/2 to 5/2 and 3 1/3 to 10/3.
• Multiply the fractions: (5/2) × (10/3) = 50/6.
• Simplify the result: 50/6 = 8 2/6 = 8 1/3.

And there you have it: 2 1/2 × 3 1/3 = 8 1/3.

When Should You Use Each Operation?

Knowing when to add, subtract, multiply, or divide mixed numbers depends on the situation. Addition and subtraction are great for combining or comparing quantities, while multiplication and division are perfect for scaling or splitting things up. Always think about what the problem is asking before deciding which operation to use.

Real-Life Applications of Mixed Numbers

Mixed numbers aren’t just for math class—they’re everywhere in real life. Here are a few examples:

• Cooking: Recipes often call for mixed numbers, like 1 1/2 cups of sugar or 2 1/4 teaspoons of salt.
• Construction: Carpenters and builders use mixed numbers to measure materials, like cutting a piece of wood that’s 5 3/8 inches long.
• Finance: Budgeting and calculating interest rates might involve mixed numbers, especially when dealing with partial amounts.
• Travel: Estimating distances or gas mileage often requires working with mixed numbers, like driving 12 1/2 miles to the store.

By understanding mixed numbers, you’ll be better equipped to tackle these kinds of problems in your daily life.

Common Mistakes to Avoid

Even the best mathematicians make mistakes sometimes. Here are a few common pitfalls to watch out for when working with mixed numbers:

• Forgetting to Simplify: Always simplify your fractions whenever possible—it makes the numbers easier to work with.
• Skipping Steps: Don’t rush through the process. Take your time and break each problem into smaller steps.
• Confusing Numerators and Denominators: Double-check that you’re putting the right numbers in the right places.
• Not Finding Common Denominators: When adding or subtracting fractions, make sure they have the same denominator before proceeding.

Avoiding these mistakes will help you get the right answers every time.

Conclusion

So, there you have it—everything you need to know about mixed numbers. From definitions to real-life applications, we’ve covered it all. Mixed numbers might seem intimidating at first, but with a little practice, they become second nature. Whether you’re cooking, building, or budgeting, mixed numbers are a valuable tool to have in your arsenal.

Now that you’ve got the basics down, why not test your skills? Try solving a few practice problems or look for examples in your daily life. And don’t forget to share this article with anyone who could benefit from it. Happy math-ing!

Daftar Isi

• What Exactly is a Mixed Number?
• Why Do Mixed Numbers Matter?
• Breaking Down the Components of a Mixed Number
• How to Convert Mixed Numbers to Improper Fractions
• Converting Improper Fractions Back to Mixed Numbers
• Adding and Subtracting Mixed Numbers
• Multiplying and Dividing Mixed Numbers
• Real-Life Applications of Mixed Numbers
• Common Mistakes to Avoid
• Conclusion