Mixed Number Definition: A Comprehensive Guide To Understanding And Mastering Fractions

Have you ever stumbled upon the term "mixed number" and wondered what it really means? Well, you’re not alone! Mixed numbers are a fundamental concept in mathematics that many people encounter throughout their academic journey. Whether you're a student trying to ace your math exams or an adult brushing up on your arithmetic skills, understanding mixed numbers is crucial. In this article, we’ll dive deep into the world of mixed numbers, breaking them down in a way that’s both easy to grasp and fun to learn!

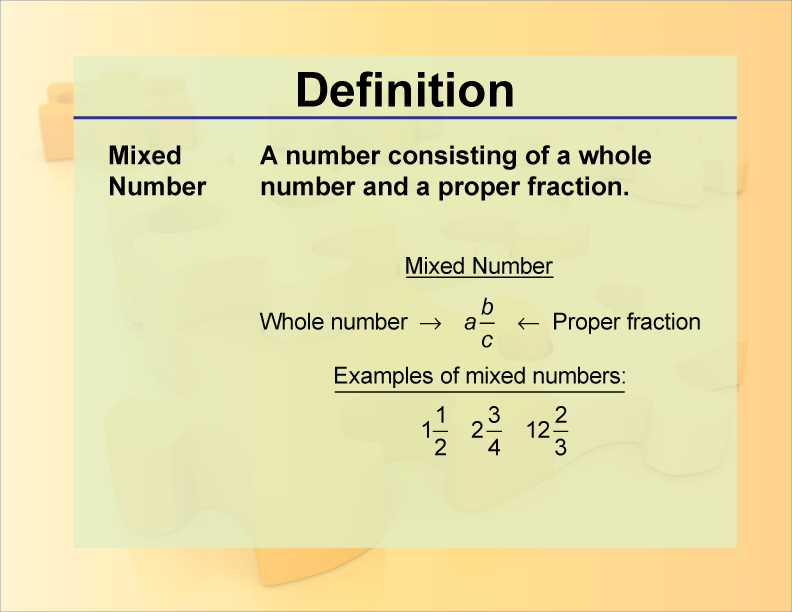
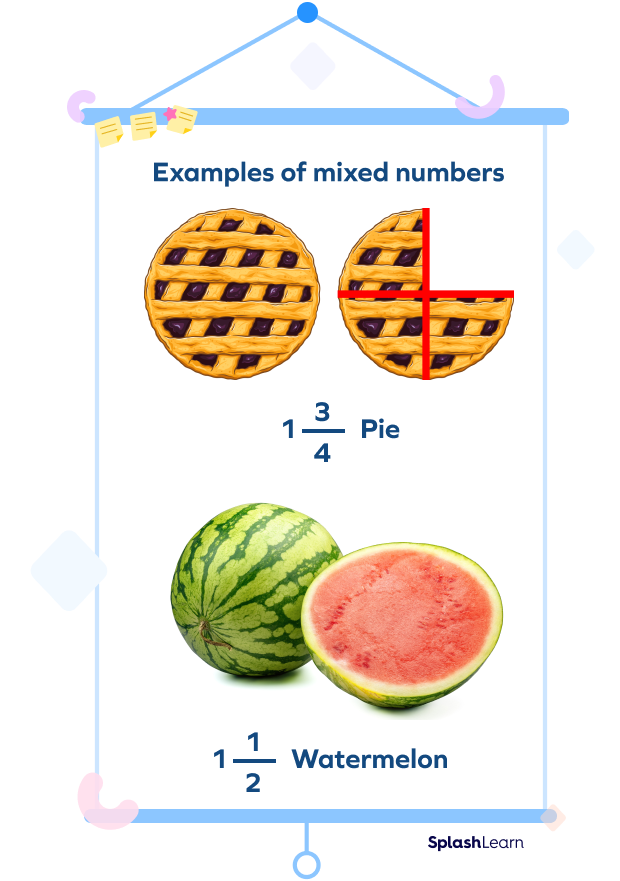
So, what exactly is a mixed number? Think of it as a combination of a whole number and a fraction. For instance, 3 1/2 is a mixed number where "3" is the whole number and "1/2" is the fraction. It’s like having three full pizzas and half of another one. Simple, right? But don’t worry if it seems a bit tricky at first—we’re here to guide you through every step of the way.

Our goal today is to make sure you leave this article with a solid understanding of mixed numbers. We’ll cover everything from their definition to real-world applications, so whether you’re solving equations or just curious about math, you’ll find something valuable here. Let’s get started!

• Susan Clark The Timeless Actress Who Stole Hearts And Made Hollywood History

Understanding Mixed Numbers: Breaking It Down

Let’s take a closer look at mixed numbers and why they’re important. At its core, a mixed number is essentially a way to express a number that has both a whole part and a fractional part. This format is incredibly useful in everyday situations, such as cooking, construction, and even budgeting.

What Makes a Number "Mixed"?

A mixed number consists of two main components: the whole number and the fraction. The whole number represents the complete units, while the fraction represents a part of a unit. For example, in the mixed number 5 3/4, "5" is the whole number, and "3/4" is the fraction.

• Whole number: The integer part of the mixed number.
• Fraction: The part of the mixed number that represents a portion of a unit.

Why Are Mixed Numbers Important?

Mixed numbers play a significant role in mathematics because they allow us to represent quantities that aren’t whole numbers more clearly. Instead of writing improper fractions (like 11/4), we can express the same value as a mixed number (2 3/4). This makes calculations easier and more intuitive.

• Is John Goodman Still Alive Unraveling The Mystery Behind The Legendary Actor

Applications in Real Life

Here are some practical examples of how mixed numbers are used in everyday life:

• Cooking: Recipes often require measurements like "1 1/2 cups of flour" or "2 1/4 teaspoons of sugar."
• Construction: Builders might need to cut wood into lengths like "6 3/8 inches."
• Finance: Budgets might include allocations like "$500 1/2 for groceries."

Converting Mixed Numbers to Improper Fractions

One of the essential skills when working with mixed numbers is converting them into improper fractions. An improper fraction is a fraction where the numerator is greater than or equal to the denominator. This conversion is useful for performing arithmetic operations like addition and subtraction.

Step-by-Step Guide

To convert a mixed number to an improper fraction, follow these steps:

1. Multiply the whole number by the denominator of the fraction.
2. Add the result to the numerator of the fraction.
3. Write the sum as the new numerator, keeping the original denominator.

For example, converting 3 2/5:

• Multiply 3 (whole number) by 5 (denominator): 3 × 5 = 15
• Add 2 (numerator): 15 + 2 = 17
• The improper fraction is 17/5

Adding and Subtracting Mixed Numbers

Adding and subtracting mixed numbers can seem intimidating at first, but with a bit of practice, it becomes second nature. The key is to break the problem into manageable steps.

Steps for Addition

Here’s how you can add two mixed numbers:

1. Convert both mixed numbers to improper fractions.
2. Find a common denominator.
3. Add the numerators, keeping the denominator the same.
4. Convert the result back to a mixed number if necessary.

For example, adding 2 1/3 and 1 2/3:

• Convert to improper fractions: 7/3 + 5/3
• Add the numerators: 7 + 5 = 12
• The result is 12/3, which simplifies to 4

Steps for Subtraction

Subtracting mixed numbers follows a similar process:

1. Convert both mixed numbers to improper fractions.
2. Find a common denominator.
3. Subtract the numerators, keeping the denominator the same.
4. Convert the result back to a mixed number if necessary.

For example, subtracting 3 1/4 from 5 3/4:

• Convert to improper fractions: 23/4 - 13/4
• Subtract the numerators: 23 - 13 = 10
• The result is 10/4, which simplifies to 2 1/2

Multiplying and Dividing Mixed Numbers

Multiplication and division of mixed numbers require converting them to improper fractions first. Once in improper fraction form, these operations become straightforward.

Multiplication

To multiply mixed numbers:

1. Convert both mixed numbers to improper fractions.
2. Multiply the numerators together and the denominators together.
3. Simplify the resulting fraction if possible.
4. Convert back to a mixed number if necessary.

For example, multiplying 2 1/2 and 1 1/3:

• Convert to improper fractions: 5/2 × 4/3
• Multiply: (5 × 4) / (2 × 3) = 20/6
• Simplify: 20/6 = 10/3
• Convert back: 3 1/3

Division

To divide mixed numbers:

1. Convert both mixed numbers to improper fractions.
2. Flip the second fraction (find its reciprocal).
3. Multiply the first fraction by the reciprocal of the second fraction.
4. Simplify the result and convert back to a mixed number if necessary.

For example, dividing 3 1/2 by 1 1/4:

• Convert to improper fractions: 7/2 ÷ 5/4
• Flip the second fraction: 7/2 × 4/5
• Multiply: (7 × 4) / (2 × 5) = 28/10
• Simplify: 28/10 = 14/5
• Convert back: 2 4/5

Common Mistakes to Avoid

When working with mixed numbers, it’s easy to make mistakes if you’re not careful. Here are a few common pitfalls to watch out for:

• Forgetting to convert to improper fractions: Always convert mixed numbers to improper fractions before performing arithmetic operations.
• Ignoring simplification: Always simplify your results to ensure accuracy.
• Misaligning denominators: Make sure the denominators are the same when adding or subtracting fractions.

Real-World Examples of Mixed Numbers

Mixed numbers aren’t just abstract concepts—they have practical applications in our daily lives. Let’s explore a few examples:

Cooking Measurements

Imagine you’re following a recipe that calls for "2 1/4 cups of flour." This is a mixed number! Understanding how to work with mixed numbers ensures you measure ingredients accurately.

Construction and Carpentry

Builders often deal with measurements like "4 3/8 inches." Knowing how to add, subtract, and convert mixed numbers helps them cut materials precisely.

Finance and Budgeting

When creating a budget, you might allocate "$500 1/2 for groceries." Mixed numbers allow for more detailed financial planning.

Conclusion: Mastering Mixed Numbers

In conclusion, mixed numbers are an essential part of mathematics with numerous real-world applications. By understanding their definition, learning how to convert them to improper fractions, and mastering arithmetic operations, you can tackle any problem involving mixed numbers with confidence.

Now it’s your turn! Share your thoughts in the comments below. Have you encountered mixed numbers in your daily life? What challenges did you face, and how did you overcome them? Don’t forget to explore other articles on our site for more math tips and tricks!

Table of Contents

• Understanding Mixed Numbers: Breaking It Down
• Why Are Mixed Numbers Important?
• Converting Mixed Numbers to Improper Fractions
• Adding and Subtracting Mixed Numbers
• Multiplying and Dividing Mixed Numbers
• Common Mistakes to Avoid
• Real-World Examples of Mixed Numbers
• Conclusion: Mastering Mixed Numbers