What Is A Mixed Number? A Deep Dive Into The World Of Fractions And Numbers
Alright folks, let’s get straight to the point. If you’re here, you’re probably wondering, “What is a mixed number?” Well, buckle up because we’re about to break it down in a way that’s easy to understand, super useful, and packed with examples. A mixed number, my friends, is a combination of a whole number and a proper fraction. Think of it as a perfect blend of two worlds—whole numbers and fractions—working together in harmony. Let’s dive deeper, shall we?
Now, you might be asking yourself why this matters. Well, mixed numbers are everywhere in real life. From cooking recipes to construction projects, they pop up in situations where you need to measure something that’s not entirely whole. Understanding them can make your life a whole lot easier, especially when you’re dealing with math problems or practical scenarios.
So, whether you’re a student trying to ace your math test, a parent helping your kid with homework, or just someone curious about numbers, this article’s got you covered. We’ll explore everything from the basics to advanced concepts, sprinkling in some fun facts and practical tips along the way. Ready? Let’s go!
Table of Contents
- What is a Mixed Number?
- A Brief History of Mixed Numbers
- Types of Numbers and Fractions
- Converting Mixed Numbers
- Real-Life Applications
- Common Mistakes to Avoid
- Solving Problems with Mixed Numbers
- Tips and Tricks for Mastery
- Frequently Asked Questions
- Conclusion
What is a Mixed Number?
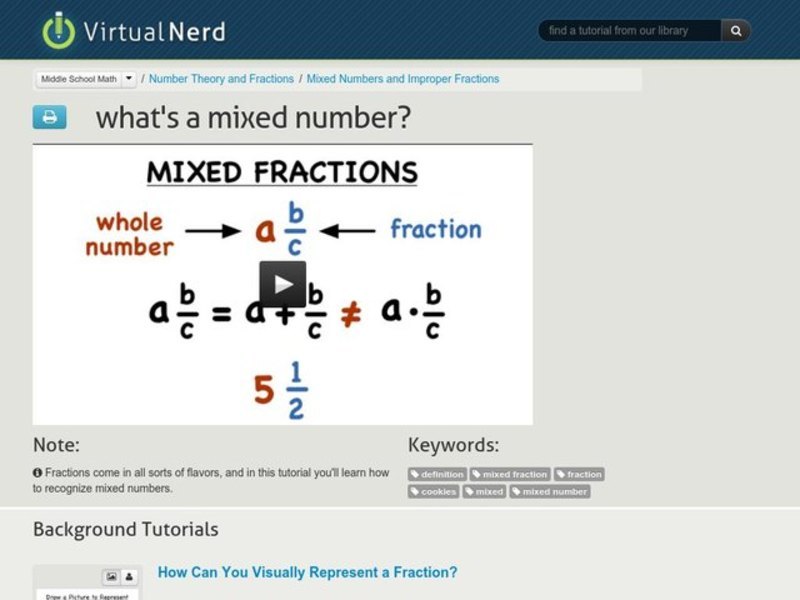
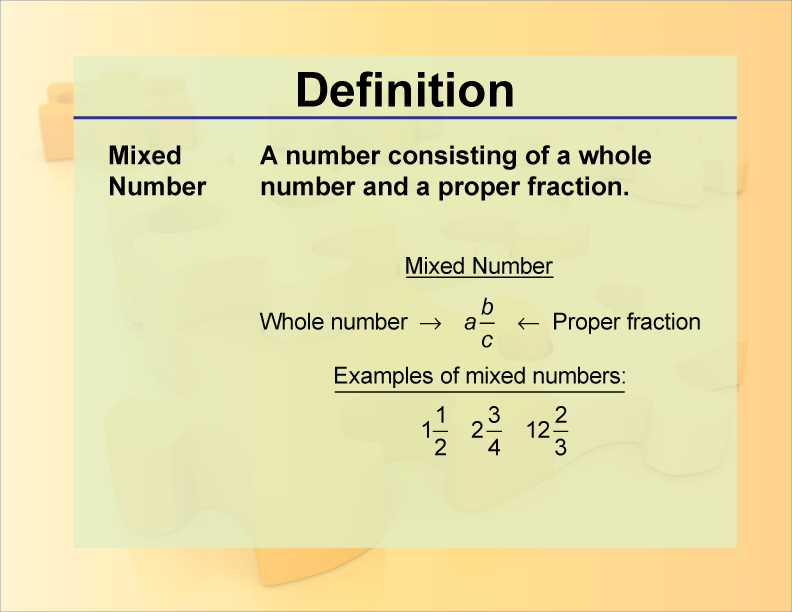
Let’s start with the basics. A mixed number, also known as a mixed fraction, is a number that consists of a whole number and a proper fraction. It’s like saying, “I’ve got 2 whole apples and half of another one.” In mathematical terms, it looks something like this: 2 1/2. The first part, 2, is the whole number, and the second part, 1/2, is the fraction.
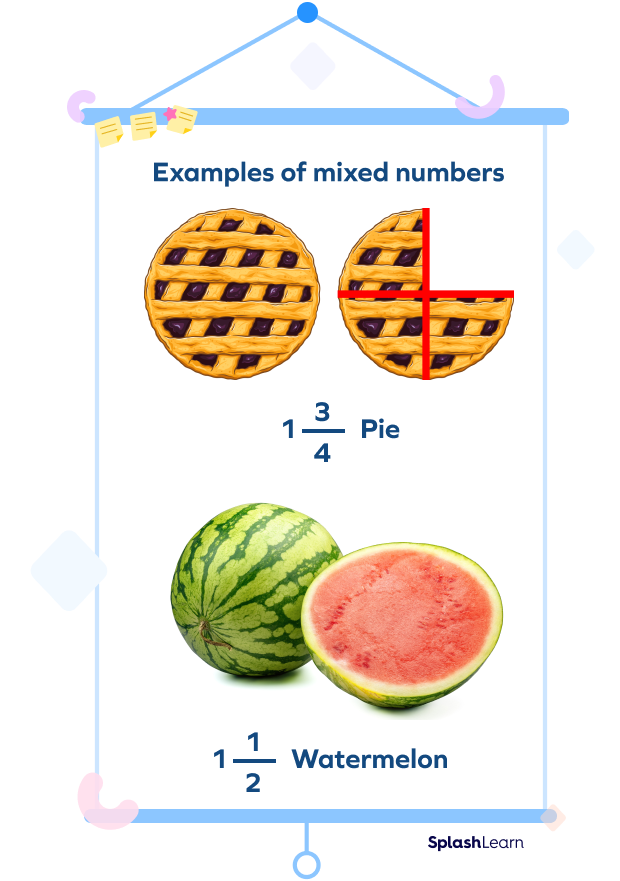
Here’s the cool thing about mixed numbers: they’re a practical way to represent quantities that aren’t entirely whole. Imagine cutting a pizza into 8 slices and eating 5 of them. You’ve got 3 slices left, but you can also say you’ve eaten 5/8 of the pizza. If you combine that with another whole pizza, you could say you’ve got 1 5/8 pizzas. See how it works?
Why Mixed Numbers Matter
Mixed numbers matter because they bridge the gap between whole numbers and fractions. They’re not just theoretical concepts—they’re tools we use in everyday life. Whether you’re dividing up resources, measuring ingredients, or calculating distances, mixed numbers make math more relatable and applicable.
A Brief History of Mixed Numbers
Believe it or not, mixed numbers have been around for centuries. Back in the day, ancient civilizations like the Egyptians and Babylonians used fractions to solve practical problems. They didn’t have calculators or fancy apps, so they relied on their wits and math skills. Mixed numbers evolved as a way to simplify complex calculations and make them more intuitive.
Fast forward to modern times, and mixed numbers are still an essential part of mathematics education. They’re taught in schools worldwide because they help students understand the relationship between whole numbers and fractions. Plus, they’re just plain useful!
Types of Numbers and Fractions
Before we dive deeper into mixed numbers, let’s take a quick look at the different types of numbers and fractions. This’ll give you a better understanding of where mixed numbers fit in the grand scheme of things.
- Whole Numbers: These are numbers like 0, 1, 2, 3, and so on. No fractions or decimals allowed here.
- Fractions: Fractions represent parts of a whole. They come in two flavors: proper fractions (e.g., 1/2) and improper fractions (e.g., 5/3).
- Mixed Numbers: As we’ve discussed, these are a combination of whole numbers and proper fractions.
Understanding these categories will help you navigate the world of numbers with confidence.
Proper vs. Improper Fractions
Now, here’s a quick breakdown of proper and improper fractions:
- Proper Fractions: These are fractions where the numerator (top number) is smaller than the denominator (bottom number). For example, 3/4 is a proper fraction.
- Improper Fractions: These are fractions where the numerator is greater than or equal to the denominator. For example, 7/3 is an improper fraction.
Why does this matter? Well, improper fractions can be converted into mixed numbers, and vice versa. We’ll cover that in the next section.
Converting Mixed Numbers
Converting mixed numbers is a crucial skill to master. Whether you’re converting them to improper fractions or the other way around, knowing how to do it will make your life a whole lot easier.
From Mixed Numbers to Improper Fractions
Here’s how you convert a mixed number to an improper fraction:
- Multiply the whole number by the denominator.
- Add the result to the numerator.
- Write the new numerator over the original denominator.
For example, let’s convert 3 2/5:
- Whole number: 3
- Denominator: 5
- Numerator: 2
Step 1: 3 × 5 = 15
Step 2: 15 + 2 = 17
Step 3: 17/5
So, 3 2/5 becomes 17/5. Easy peasy!
From Improper Fractions to Mixed Numbers
Converting an improper fraction to a mixed number is just as simple:
- Divide the numerator by the denominator.
- The quotient becomes the whole number.
- The remainder becomes the new numerator, and the denominator stays the same.
For example, let’s convert 11/4:
- Numerator: 11
- Denominator: 4
Step 1: 11 ÷ 4 = 2 remainder 3
Step 2: Whole number = 2
Step 3: Remainder = 3, Denominator = 4
So, 11/4 becomes 2 3/4. Cool, right?
Real-Life Applications
Mixed numbers aren’t just theoretical concepts—they’re super useful in real life. Let’s explore a few examples:
Cooking and Baking
Ever tried following a recipe that calls for 1 1/2 cups of flour? That’s a mixed number in action. Understanding how to measure and convert mixed numbers will make your cooking adventures a whole lot smoother.
Construction and Engineering
In construction, measurements often involve mixed numbers. For instance, a beam might be 8 3/4 feet long. Knowing how to work with mixed numbers ensures accuracy and precision.
Finance and Budgeting
When dealing with money, mixed numbers can help you split costs or calculate portions. For example, if you’re splitting a $25 bill among 4 people, each person pays $6 1/4. Makes sense, right?
Common Mistakes to Avoid
Even the best of us make mistakes when working with mixed numbers. Here are a few pitfalls to watch out for:
- Improper Conversion: Double-check your math when converting between mixed numbers and improper fractions. A small error can throw off your entire calculation.
- Ignoring Simplification: Always simplify fractions whenever possible. For example, 4 2/6 can be simplified to 4 1/3.
- Forgetting Units: When working with measurements, don’t forget to include units like inches, cups, or dollars. It’s all about clarity!
Solving Problems with Mixed Numbers
Now that you’ve got the basics down, let’s tackle some real-world problems involving mixed numbers. We’ll use examples to illustrate how to solve them step by step.
Example 1: Adding Mixed Numbers
Problem: Add 2 1/3 and 1 2/5.
Solution:
- Convert both mixed numbers to improper fractions.
- Find a common denominator.
- Add the fractions.
- Convert back to a mixed number if needed.
Step 1: 2 1/3 = 7/3, 1 2/5 = 7/5
Step 2: Common denominator = 15
Step 3: (7/3 × 5/5) + (7/5 × 3/3) = 35/15 + 21/15 = 56/15
Step 4: 56 ÷ 15 = 3 remainder 11, so 56/15 = 3 11/15
Answer: 3 11/15
Example 2: Subtracting Mixed Numbers
Problem: Subtract 4 3/8 from 6 1/4.
Solution:
- Convert both mixed numbers to improper fractions.
- Find a common denominator.
- Subtract the fractions.
- Convert back to a mixed number if needed.
Step 1: 6 1/4 = 25/4, 4 3/8 = 35/8
Step 2: Common denominator = 8
Step 3: (25/4 × 2/2) - 35/8 = 50/8 - 35/8 = 15/8
Step 4: 15 ÷ 8 = 1 remainder 7, so 15/8 = 1 7/8
Answer: 1 7/8
Tips and Tricks for Mastery
Becoming a pro at mixed numbers takes practice, but here are a few tips to help you along the way:
- Practice Regularly: The more you practice, the better you’ll get. Try solving problems every day to sharpen your skills.
- Use Visual Aids: Diagrams and models can help you visualize mixed numbers and understand them better.
- Memorize Key Concepts: Knowing the steps for converting and solving mixed numbers will save you time and effort.
Frequently Asked Questions
Q: Can mixed numbers be negative?
A: Absolutely! A negative mixed number is just a mixed number with a negative sign. For example, -3 1/2.
Q: Are mixed numbers always easier to work with than improper fractions?
A: Not necessarily. It depends on the situation. Sometimes improper fractions are more convenient, especially when multiplying or dividing.
Q: How do I compare mixed numbers?
A: Convert them to improper fractions, find a common denominator, and compare the numerators.
Conclusion
And there you have it,